Solution to a pair of linear, two-variable, Diophantine equations with coprime coefficients from balancing and Lucas-balancing numbers

R. K. Davala
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 29, 2023, Number 3, Pages 495–502
DOI: 10.7546/nntdm.2023.29.3.495-502
Full paper (PDF, 222 Kb)

Details

Authors and affiliations

R. K. Davala
VIT-AP University, Amaravati, Andhra Pradesh, India

Abstract

Let B_n and C_n be the n-th balancing and Lucas-balancing numbers, respectively. We consider the Diophantine equations ax+by=\frac{1}{2}(a-1)(b-1) and 1+ax+by=\frac{1}{2}(a-1)(b-1) for (a,b) \in \{(B_n,B_{n+1}),(B_{2n-1},B_{2n+1}), (B_n,C_n),(C_n,C_{n+1})\} and present the non-negative integer solutions of the Diophantine equations in each case.

Keywords

  • Balancing numbers
  • Lucas-balancing numbers
  • Diophantine equation

2020 Mathematics Subject Classification

  • 11B39
  • 11B37

References

  1. Behera, A., & Panda, G. K. (1999). On the square roots of triangular numbers. The Fibonacci Quarterly, 37(2), 98–105.
  2. Beiter, M. (1964). The midterm coefficient of the cyclotomic polynomial 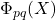\Phi_{pq}(X). American Mathematical Monthly, 71, 769–770.
  3. Chu, H. V. (2020). Representation of \frac{1}{2}(F_n-1)(F_{n+1}-1) and \frac{1}{2}(F_n-1)(F_{n+2}-1). The Fibonacci Quarterly, 58(4), 334–339.
  4. Davala, R. K. (2015). On convolution and binomial sums of balancing and Lucas-balancing numbers. International Journal of Mathematical Sciences and Engineering Applications, 8(5), 77–83.
  5. Davala, R. K. (2018). Algebraic and geometric aspects of some binary recurrence
    sequences. Ph.D. Thesis, National Institute of Technology, Rourkela, India.
  6. Davala, R. K., & Panda, G. K. (2015). On sum and ratio formulas for balancing numbers. The Journal of the Indian Mathematical Society, 82(2), 23–32.
  7. Davala, R. K., & Panda, G. K. (2016). On sum and ratio formulas for balancing-like sequences. Notes on Number Theory and Discrete Mathematics, 22(3), 45–53.
  8. Davala, R. K., & Panda, G. K. (2019). On sum and ratio formulas for Lucas-balancing numbers. Palestine Journal of Mathematics, 8(2), 200–206.
  9. Frontczak, R. (2018). Sums of balancing and Lucas-balancing numbers with binomial coefficients. International Journal of Mathematical Analysis, 12, 585–594.
  10. Panda, G. K. (2009). Some fascinating properties of balancing numbers. Congressus Numerantium, 194, 265–271.
  11. Ray, P. K. (2009). Balancing and cobalancing numbers. Ph.D. Thesis, National Institute of Technology, Rourkela, India.
  12. Ray, P. K. (2015). Balancing and Lucas-balancing sums by matrix methods. Mathematical Reports, 17(2), 225–233.
  13. Soykan, Y. (2021). A study on generalized balancing numbers. Asian Journal of Advanced Research and Reports, 15(5), 78–100.

Manuscript history

  • Received: 10 March 2023
  • Revised: 28 June 2023
  • Accepted: 13 July 2023
  • Online First: 15 July 2023

Copyright information

Ⓒ 2023 by the Author.
This is an Open Access paper distributed under the terms and conditions of the Creative Commons Attribution 4.0 International License (CC BY 4.0).

Related papers

Cite this paper

Davala, R. K. (2023). Solution to a pair of linear, two-variable, Diophantine equations with coprime coefficients from balancing and Lucas-balancing numbers. Notes on Number Theory and Discrete Mathematics, 29(3), 495-502, DOI: 10.7546/nntdm.2023.29.3.495-502.

Comments are closed.