Efruz Özlem Mersin
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 29, 2023, Number 2, Pages 389–401
DOI: 10.7546/nntdm.2023.29.2.389-401
Full paper (PDF, 213 Kb)
Details
Authors and affiliations
Efruz Özlem Mersin ![]()
![]()
Department of Mathematics, Faculty of Science and Arts,
Aksaray University, Aksaray, Turkey
Abstract
The aim of this paper is to introduce the hybrid form of the hyperbolic Horadam function and to investigate some of its properties such as the generating function. Another aim is to define hyperbolic Horadam hybrid sine and cosine functions and their symmetrical forms. For newly defined functions, some properties such as the recursive relations, derivatives, Cassini and De Moivre type identities are examined. In addition, the quasi-sine Horadam hybrid function and three-dimensional Horadam hybrid spiral are defined.
Keywords
- Hyperbolic functions
- Hybrid numbers
- Horadam numbers
2020 Mathematics Subject Classification
- 11B37
- 11B39
- 11K31
- 11Y55
References
- Ait-Amrane, N., Belbachir, H., & Tan, E. (2022). On generalized Fibonacci and Lucas hybrid polynomials. Turkish Journal of Mathematics, 46(6), 2069–2077.
- Alp, Y., & Koçer, E. G. (2021). Hybrid Leonardo numbers. Chaos, Solitons & Fractals, 150, Article 111128.
- Bahşi, M., & Solak, S. (2019). Hyperbolic Horadam functions. Gazi University Journal of Science, 32(3), 956–965.
- Edson, M., & Yayenie, O. (2009). A new generalization of Fibonacci sequences and extended Binet’s formula. Integers, 9, 639–654.
- Falcón, S., & Plaza, Á. (2007). On the Fibonacci k-numbers. Chaos, Solitons & Fractals, 32(5), 1615–1624.
- Falcón, S., & Plaza, Á. (2008). The k-Fibonacci hyperbolic functions. Chaos, Solitons & Fractals, 38(2), 409–420.
- Horadam, A. F. (1965). Basic properties of a certain generalized sequence of numbers. The Fibonacci Quarterly, 3(3), 161–176.
- Kılıç, E., & Tan, E. (2009). More general identities involving the terms of
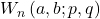 . Ars Combinatoria, 93, 459–461.
. Ars Combinatoria, 93, 459–461. - Kılıç, N. (2022). Introduction to k-Horadam hybrid numbers. Kuwait Journal of Science, 49(4), DOI: 10.48129/kjs.14929.
- Kızılateş, C. (2022). A note on Horadam hybrinomials. Fundamental Journal of
Mathematics and Applications, 5(1), 1–9. - Koçer, E. G., Tuglu, N., & Stakhov, A. (2008). Hyperbolic functions with second order recurrence sequences. Ars Combinatoria, 88, 65–81.
- Koshy, T. (2001). Fibonacci and Lucas Numbers with Applications. Pure and Applied Mathematics, A Wiley-Interscience Series of Texts, Monographs and Tracts, New York: Wiley.
- Özdemir, M. (2018). Introduction to hybrid numbers. Advances in Applied Clifford Algebras, 28, 1–32.
- Özimamoglu, H. (2023). A new generalization of Leonardo hybrid numbers with q-integers. Indian Journal of Pure and Applied Mathematics, DOI: 10.1007/s13226-023-00365-7.
- Pandir, Y., & Ulusoy, H. (2013). New generalized hyperbolic functions to find new exact solutions of the nonlinear partial differential equations. Journal of Mathematics, 2013, Article 201276.
- Şentürk, T. C., Bilgici, G., Daşdemir, A., & Ünal, Z. (2020). A study on Horadam hybrid numbers. Turkish Journal of Mathematics, 44, 1212–1221.
- Spinadel, V. W. (1998). From the Golden Mean to Chaos. Nueva Libreria. (Second Edition, Nobuko 2004).
- Stakhov, A. P. (2006). Gazale formulas, a new class of the hyperbolic Fibonacci and Lucas functions and the improved method of the “golden” cryptography. Moscow: Academy of Trinitarism, 1, Article 77-6567.
- Stakhov, A. P., & Tkachenko, I.S. (1993). Hyperbolic Fibonacci trigonometry. Reports of Ukraine Academy of Sciences, 208(7), 9–14.
- Stakhov, A. P., & Rozin, B. (2005). On a new class of hyperbolic functions. Chaos, Solitons & Fractals, 23(2), 379–389.
- Stakhov, A. P., & Rozin, B. (2005). The golden shofar. Chaos, Solitons & Fractals, 26(3), 677–684.
- Stakhov, A. P., & Rozin, B. (2006). The continuous functions for the Fibonacci and Lucas p-numbers. Chaos, Solitons & Fractals, 28(4), 1014–1025.
- Szynal-Liana, A. (2018). The Horadam hybrid numbers. Discussiones Mathematicae General Algebra and Applications, 38(1), 91–98.
- Szynal-Liana, A., & Włoch, I. (2018). On Pell and Pell–Lucas Hybrid Numbers.
Commentationes Mathematicae, 58(1-2), 11–17. - Szynal-Liana, A., & Włoch, I. (2019). On Jacopsthal and Jacopsthal-Lucas hybrid numbers. Annales Mathematicae Silesianae, 33, 276–283.
- Szynal-Liana, A., & Włoch, I. (2020). Introduction to Fibonacci and Lucas hybrinomials. Complex Variables and Elliptic Equations, 65(10), 1736–1747.
- Tan, E., & Ait-Amrane, N. R. (2022). On a new generalization of Fibonacci hybrid numbers. Indian Journal of Pure and Applied Mathematics, 54(2), 428–438.
- Taşcı, D., & Sevgi, E. (2021). Some properties between Mersenne, Jacobsthal and Jacobsthal–Lucas hybrid numbers. Chaos, Solitons & Fractals, 146, 110862.
- Yayenie, O. ( 2011). A note on generalized Fibonacci sequences. Applied Mathematics and Computation, 217(12), 5603–5611.
- Yazlik, Y., & Taskara, N. (2012). A note on generalized k-Horadam sequence. Computers & Mathematics with Applications, 63(1), 36–41.
Manuscript history
- Received: 18 November 2022
- Revised: 10 May 2023
- Accepted: 20 May 2023
- Online First: 23 May 2023
Copyright information
![]() Ⓒ 2023 by the Author.
Ⓒ 2023 by the Author.
This is an Open Access paper distributed under the terms and conditions of the Creative Commons Attribution 4.0 International License (CC BY 4.0).
Related papers
Cite this paper
Mersin, E. Ö. (2023). Hyperbolic Horadam hybrid functions. Notes on Number Theory and Discrete Mathematics, 29(2), 389-401, DOI: 10.7546/nntdm.2023.29.2.389-401.


