Half self-convolution of the k-Fibonacci sequence

Sergio Falcon
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 26, 2020, Number 3, Pages 96–106
DOI: 10.7546/nntdm.2020.26.3.96-106
Full paper (PDF, 202 Kb)

Details

Authors and affiliations

Sergio Falcon
Department of Mathematics, University of Las Palmas de Gran Canaria
Campus de Tafira, 35017 – Las Palmas de Gran Canaria, Spain

Abstract

We say the -Fibonacci numbers and are equidistant if and then we study some properties of these pairs of numbers. As a main result, we look for the formula to find the generating function of the product of the equidistant numbers, their sums and their binomial transforms. Next we apply this formula to some simple cases but more common than the general. In particular, we define the half self-convolution of the -Fibonacci and -Lucas sequences. Finally, we study the sum of these new sequences, their recurrence relations, and their generating functions.

Keywords

• -Fibonacci and -Lucas numbers
• Binet identity
• Generating function
• Convolution
• Binomial transform

2010 Mathematics Subject Classification

• 11B37
• 11B39
• 11B65

References

1. Falcon, S. (2011) On the -Lucas numbers, Int. J. Contemp. Math. Sciences, 6(21), 1039–1050.
2. Falcon, S. (2012) On the -Lucas numbers of arithmetic indexes, Applied Mathematics, 3, 1202–1206.
3. Falcon, S. (2014). On the Generating Functions of the Powers of the -Fibonacci Numbers, Scholars Journal of Engineering and Technology (SJET), 2(4C), 669–675.
4. Falcon, S., & Plaza, A. (2007). On the Fibonacci -numbers, Chaos, Solit. & Fract., 32(5), 1615–1624.
5. Falcon, S., & Plaza, A. (2007). The -Fibonacci sequence and the Pascal 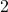-triangle, Chaos, Solit. & Fract., 33(1), 38–49.
6. Sloane, N. J. A., editor. The On-Line Encyclopedia of Integer Sequences, Available online at: https://oeis.org.
7. Wilf, H. S. (1994). Generating functionology, Ed. Academic Press Inc., Available online at: http://www.math.upenn.edu/˜wilf/DownldGF.html.