On the equation f(n2 − Dnm + m2) = f 2(n) − Df(n)f(m) + f 2(m)

B. M. Phong and R. B. Szeidl
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 28, 2022, Number 2, Pages 240–251
DOI: 10.7546/nntdm.2022.28.2.240-251
Full paper (PDF, 192 Kb)

Details

Authors and affiliations

B. M. Phong
Department of Computer Algebra, University of Eötvös Loránd
1117 Budapest, Hungary

R. B. Szeidl
Department of Computer Algebra, University of Eötvös Loránd
1117 Budapest, Hungary

Abstract

We give all solutions f:\Bbb N \to \Bbb C of the functional equation

    \[f(n^2-Dnm+m^2)=f^2(n)-Df(n)f(m)+f^2(m),\]

where D\in\{1,2\}.

Keywords

  • Arithmetical function
  • Functional equation
  • Dirichlet character

2020 Mathematics Subject Classification

  • 11A07
  • 11A25
  • 11N25
  • 11N64

References

  1. De Koninck, J.-M., Kátai, I., & Phong, B. M. (1997). A new characteristic of the identity function. Journal of Number Theory, 63(2), 325–338.
  2. Khanh, B. M. M. (2021). Characterization of the identity function with an equation function. Annales Universitatis Scientiarium Budapestinensis de Rolando Eötvös Nominatae. Sectio Computatorica, 52, 195–216.
  3. Park, P.-S. (in press). Multiplicative functions commutable with binary quadratic forms 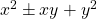x^2 \pm xy + y^2. Bulletin of the Korean Mathematical Society.
  4. Phong, B. M. (2006). A characterization of the identity function with the equation of Hosszú type. Publicationes Mathematicae Debrecen, 69(1–2), 219–226.
  5. Phong, B. M. (2016). A characterization of identity with function equation II. Acta Mathematica Hungarica, 148(2), 450–465.
  6. Phong, B. M., & Szeidl, R. B. (2021). On the equation 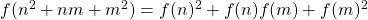f(n^2+nm+m^2)=f(n)^2+f(n)f(m)+f(m)^2. Annales Universitatis Scientiarium Budapestinensis de Rolando Eötvös Nominatae. Sectio Computatorica, 52, 255–278.
  7. Spiro, C. (1992). Additive uniqueness set for arithmetic functions. Journal of Number Theory, 42, 232–246.

Manuscript history

  • Received: 29 August 2021
  • Revised: 23 April 2022
  • Accepted: 28 April 2022
  • Online First: 29 April 2022

Related papers

Cite this paper

Phong, B. M., & Szeidl, R. B. (2022). On the equation f(n2 − Dnm + m2) = f2(n) − Df(n)f(m) + f2(m). Notes on Number Theory and Discrete Mathematics, 28(2), 240-251, DOI: 10.7546/nntdm.2022.28.2.240-251.

Comments are closed.