Nguyen Viet Dung and Luu Ba Thang
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 29, 2023, Number 1, Pages 24–29
DOI: 10.7546/nntdm.2023.29.1.24-29
Full paper (PDF, 216 Kb)
Details
Authors and affiliations
Nguyen Viet Dung 
Department of Mathematics and Informatics, Hanoi National University of Education
36 Xuan Thuy, Cau Giay, Hanoi, Vietnam
Luu Ba Thang
Department of Mathematics and Informatics, Hanoi National University of Education
36 Xuan Thuy, Cau Giay, Hanoi, Vietnam
Abstract
We say that a positive integer 
is special number of degree 
if for every integer 
, there exist nonzero integers 
such that 
In this paper, we investigate some necessary conditions on for existing a special number of degree 
Keywords
- Representation of integers
2020 Mathematics Subject Classification
- 11E25
References
- Dung, N. V., & Thang, L. B. (2021). The number
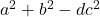revisited. Journal of Integer Sequences, 24, Article 21.9.3. - Nathason, M. B. (2021). Combinatorial and Additive Number Theory IV:CANT. Springer, New York, USA.
- Nowicki, A. (2015). The number
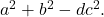Journal of Integer Sequences, 18, Article 15.2.3. - Prugsapitak S., & Thongngam, N. (2021). Representation of integers of the form
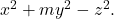Journal of Integer Sequences, 24, Article 24.7.7. - Prugsapitak S., & Thongngam, N. (2022). Representation of integers of the form
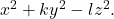Journal of Integer Sequences, 25, Article 22.8.5. - Sierpiński, W. (1988). Elementary Theory of Numbers. North-Holland.
Manuscript history
- Received: 24 May 2022
- Revised: 28 January 2023
- Accepted: 6 February 2023
- Online First: 8 February 2023
Copyright information
Ⓒ 2023 by the Authors.
This is an Open Access paper distributed under the terms and conditions of the Creative Commons Attribution 4.0 International License (CC BY 4.0).
Related papers
Cite this paper
Dung, N. V., & Thang, L. B. (2023). A note on the number an+ bn – dcn. Notes on Number Theory and Discrete Mathematics, 29(1), 24-29, DOI: 10.7546/nntdm.2023.29.1.24-29.
