On the set of Set(n)’s

Krassimir T. Atanassov
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 30, 2024, Number 3, Pages 590–594
DOI: 10.7546/nntdm.2024.30.3.590-594
Full paper (PDF, 175 Kb)

Details

Authors and affiliations

Krassimir T. Atanassov
Department of Bioinformatics and Mathematical Modelling, Institute of Biophysics and Biomedical Engineering, Bulgarian Academy of Sciences
105 Acad. G. Bonchev Str., 1113 Sofia, Bulgaria

Abstract

The set of ‘s for natural numbers is constructed. For this set it is proved that it is a commutative semi-group. The conditions for which it is a monoid are given.

Keywords

• Monoid
• Natural number
• 

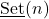
2020 Mathematics Subject Classification

• 11A25

References

1. Atanassov, K. (2020). Objects generated by an arbitrary natural number. Notes on Number Theory and Discrete Mathematics, 26(4), 57–62.
2. Atanassov, K. (2022). Objects generated by an arbitrary natural number. Part 2: Modal aspect. Notes on Number Theory and Discrete Mathematics, 28(3), 558–563.
3. Atanassov, K. (2023). Objects generated by an arbitrary natural number. Part 3: Standard modal-topological aspect. Notes on Number Theory and Discrete Mathematics, 29(1), 171–180.
4. Atanassov, K. (2023). Objects generated by an arbitrary natural number. Part 4: New aspects. Notes on Number Theory and Discrete Mathematics, 29(3), 589–597.
5. Sandor, J., & Crstici, B. (2005). Handbook of Number Theory. II. Springer Verlag, Berlin

Manuscript history

• Received: 10 June 2024
• Revised: 29 September 2024
• Accepted: 16 October 2024
• Online First: 22 October 2024

Copyright information

Ⓒ 2024 by the Author.
This is an Open Access paper distributed under the terms and conditions of the Creative Commons Attribution 4.0 International License (CC BY 4.0).