On the distribution of powerful and r-free lattice points

Sunanta Srisopha and Teerapat Srichan
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 30, 2024, Number 2, Pages 427–435
DOI: 10.7546/nntdm.2024.30.2.427-435
Full paper (PDF, 246 Kb)

Details

Authors and affiliations

Sunanta Srisopha
Department of Mathematics, Faculty of Science,
Valaya Alongkorn Rajabhat University under the Royal Patronage Pathum Thani Province,
Pathumthani 13180, Thailand

Teerapat Srichan
Department of Mathematics, Faculty of Science,
Kasetsart University
Bangkok 10900, Thailand

Abstract

Let . For , a lattice point is powerful if and only if is a powerful number, where is the greatest common divisor function. In this paper, we count the number of the ordered pairs ,  such that the lattice point is powerful. Moreover, we study -free lattice points analogues of powerful lattice points.

Keywords

• Greatest common divisor
• Piatetski-Shapiro sequence
• -free lattice points

2020 Mathematics Subject Classification

• 11N37
• 11N45

References

1. Akbal, Y. (2017). Friable values of Piatetski-Shapiro sequences. Proceedings of the American Mathematical Society, 145(10), 4255–4268.
2. Baker, R., Banks, W., Brudern, J., Shparlinski, I., & Weingartner, A. (2013). Piatetski-Shapiro sequences. Acta Arithmetica, 1(157), 37–68.
3. Cao, X., & Zhai, W. (1998). The distribution of square-free numbers of the form 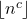. Journal de théorie des nombres de Bordeaux, 10(2), 287–299.
4. Deshouillers, J. M. (2019). A remark on cube-free numbers in Segal–Piatestki-Shapiro sequences. Hardy–Ramanujan Journal, 41, 127–132.
5. Dimitrov, S. I. (2022). Exponential sums over Piatetski-Shapiro primes in arithmetic progressions. Preprint. arXiv:2211.10928.
6. Dimitrov, S. I. (2022). Primes of the form 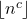with square-free . Preprint. arXiv:2207.09808.
7. Dimitrov, S. I. (2022). A Bombieri–Vinogradov type result for exponential sums over Piatetski-Shapiro primes. Lithuanian Mathematical Journal, 62(4), 435–446.
8. Guo, V. Z., Li, J., & Zhang, M. (2023). Piatetski-Shapiro primes in arithmetic progressions. The Ramanujan Journal, 60(3), 677–692.
9. Guo, V. Z., & Qi, J. (2022). A Generalization of Piatetski-Shapiro Sequences. Taiwanese Journal of Mathematics, 26(1), 33–47.
10. Ivić. A, (1985). The Theory of the Riemann Zeta Function. Wiley, New York.
11. Li, J., Zhang, M., & Xue, F. (2022). An additive problem over Piatetski-Shapiro primes and almost-primes. The Ramanujan Journal, 57(4), 1307–1333.
12. Liu, K., Shparlinski, I. E., & Zhang, T. (2017). Squares in Piatetski-Shapiro sequences. Acta Arithmetica, 181, 239–252.
13. Ma, S. (2022). On the distribution of k-full lattice points in . AIMS Mathematics, 7(6), 10596–10608.
14. Pyatetskii-Shapiro, I. I. (1953). On the distribution of prime numbers in sequences of the form ⌊f(n)⌋. Matematicheskii Sbornik, 75(3), 559–566.
15. Rieger, G. (1978). Remark on a paper of Stux concerning squarefree numbers in non-linear sequences. Pacific Journal of Mathematics, 78(1), 241–242.
16. Srichan, T. (2021). On the distribution of (k, r)-integers in Piatetski-Shapiro sequences. Czechoslovak Mathematical Journal, 71(4), 1063–1070.
17. Srichan, T., & Tangsupphathawat, P. (2020). Square-full numbers in Piatetski-Shapiro sequences. Annales mathématiques du Québec, 44, 385–391.
18. Stux, I. (1975). Distribution of squarefree integers in non-linear sequences. Pacific Journal of Mathematics, 59(2), 577–584.
19. Wang, H., & Zhang, Y. (2023). On the divisor function over Piatetski-Shapiro sequences. Czechoslovak Mathematical Journal, 73(2), 613–620.
20. Zhang, M., & Li, J. (2017). Distribution of cube-free numbers with form 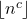. Frontiers of Mathematics in China, 12(6), 1515–1525.

Manuscript history

• Received: 11 April 2023
• Revised: 6 June 2024
• Accepted: 14 June 2024
• Online First: 20 June 2024

Copyright information

Ⓒ 2024 by the Authors.
This is an Open Access paper distributed under the terms and conditions of the Creative Commons Attribution 4.0 International License (CC BY 4.0).