Ioana Petkova
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 27, 2021, Number 4, Pages 104–115
DOI: 10.7546/nntdm.2021.27.4.104-115
Full paper (PDF, 284 Kb)
Details
Authors and affiliations
Ioana Petkova ![]()
Faculty of Mathematics and Informatics, Sofia University
Sofia, Bulgaria
Abstract
In this paper we give representations for the coefficients of the Maclaurin series for ![]() and its reciprocal (where
and its reciprocal (where ![]() is Euler’s Gamma function) with the help of a differential operator
is Euler’s Gamma function) with the help of a differential operator ![]() , the exponential function and a linear functional
, the exponential function and a linear functional ![]() (in Theorem 3.1). As a result we obtain the following representations for
(in Theorem 3.1). As a result we obtain the following representations for ![]() (in Theorem 3.2):
(in Theorem 3.2):
![Rendered by QuickLaTeX.com \begin{align*} \Gamma(z+1) & = \big(e^{-u(x)}e^{-z\mathfrak{D}}[e^{u(x)}]\big)^{*}, \\ \big(\Gamma(z+1)\big)^{-1} & = \big(e^{u(x)}e^{-z\mathfrak{D}}[e^{-u(x)}]\big)^{*}. \end{align*}](https://nntdm.net/wp-content/ql-cache/quicklatex.com-8e65fd7c44f1641db0a3814abcb8e7ab_l3.png)
Theorem 3.1 and Theorem 3.2 are our main results. With the help of the first theorem we give our approach for finding the coefficients of Maclaurin series for ![]() and its reciprocal in an explicit form.
and its reciprocal in an explicit form.
Keywords
- Gamma function
- Zeta function
- Euler’s constant
- Maclaurin series
2020 Mathematics Subject Classification
- 33B15
- 11M06
- 30D10
- 30D30
- 30K05
- 41A58
References
- Abramowitz, M. & Stegun, I. A. (1972). Handbook of Mathematical Functions, Tenth Printing, Washington.
- Choi, J., & Srivastava, H. M. (2000). Evaluation of higher-order derivatives of the Gamma function. University of Belgrade Publikacije Elektrotehnickog Fakulteta, Ser. Mat. 11 (2000), 9–18.
- Fekih-Ahmed, L. (2014). On the Power Series Expansion of the Reciprocal Gamma Function. Preprint. Retrieved from: https://hal.archives-ouvertes.fr/hal-01029331v4, 9–18.
- Kono, K. (2017). Series Expansion of Gamma Function & the Reciprocal. Retrieved from: https://fractional-calculus.com/series_expansion_gamma_reciprocal.pdf, Formula 12.1.0 (Masayuki Ui).
- Luke, Y. L. (1980). Special Mathematical Functions and Their Approximations, Mir Publishing House, Moscow (in Russian).
- Nielsen, N. (1906). Handbuch der theorie der gammafunktion, Teubner, Leipzig, 40–43.
- Olver, F. W. J. (1997). Asymptotics and Special Functions, A K Peters, Wellesley, Massachusetts.
- Sakata, M. (2017). Taylor series for the reciprocal Gamma function and multiple zeta values Proceedings of the Japan Academy, Ser. A, Mathematical Sciences, 93, 47.
- Wikipedia contributors. (2021, November 30). Gamma function. Wikipedia, The Free Encyclopedia. Retrieved from: https://en.wikipedia.org/w/index.php?title=Gamma_function&oldid=1057910449#General.
Related papers
- Petkova, I. (2024). Powers of the operator
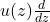 and their connection with some combinatorial numbers. Notes on Number Theory and Discrete Mathematics, 30(4), 716-734.
and their connection with some combinatorial numbers. Notes on Number Theory and Discrete Mathematics, 30(4), 716-734.
Cite this paper
Petkova, I. (2021). Series expansion of the Gamma function and its reciprocal. Notes on Number Theory and Discrete Mathematics, 27(4), 104-115, DOI: 10.7546/nntdm.2021.27.4.104-115.


