Nurettin Irmak, Zafer Şiar and Refik Keskin
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 25, 2019, Number 4, Pages 96–101
DOI: 10.7546/nntdm.2019.25.4.96-101
Full paper (PDF, 135 Kb)
Details
Authors and affiliations
Nurettin Irmak ![]()
Department of Mathematics, Ömer Halisdemir University Niğde, Turkey
Zafer Şiar ![]()
Department of Mathematics, Bingöl University Bingöl, Turkey
Refik Keskin ![]()
Department of Mathematics, Sakarya University Sakarya, Turkey
Abstract
In this paper, we solve the equations
![]()
![]()
![]()
![]()
for ![]() and a natural number
and a natural number ![]() . It is shown that only the equation
. It is shown that only the equation ![]() has a finite number of solutions. The others have infinitely many solutions.
has a finite number of solutions. The others have infinitely many solutions.
Keywords
- Fibonacci number
- Lucas number
- Rank
- Recurrences
2010 Mathematics Subject Classification
- 11B39
- 11D61
- 11B37
References
- Bugeaud, Y., Mignotte, M. & Siksek, S. (2006). Classical and modular approaches to exponential Diophantine equations I. Fibonacci and Lucas perfect powers, Annals of Mathematics, 163 (3), 969–1018.
- Carlitz, L. (1964). A note on Fibonacci numbers,The Fibonacci Quarterly, 2 (1), 15–28.
- Farrokhi, D. G. M. (2007). Some remarks on the equation
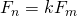 in Fibonacci numbers,Journal of Integer Sequences, 10, Article 07.5.7.
in Fibonacci numbers,Journal of Integer Sequences, 10, Article 07.5.7. - Keskin, R. & Demirtürk Bitim, B. (2011). Fibonacci and Lucas congruences and their applications, Acta Mathematica Sinica (English Series), 27(4), 725–736.
- Koshy, T. (2001). Fibonacci and Lucas Numbers with Applications, John Wiley and Sons, Proc., New York-Toronto.
- Luca, F., & Szalay, L. (2009). Lucas Diophantine Triples,Integers, 9, 441–457.
Related papers
- Nagaraja, K. M., & Dhanya, P. (2020). Identities on generalized Fibonacci and Lucas numbers. Notes on Number Theory and Discrete Mathematics, 26 (3), 189-202, doi: 10.7546/nntdm.2020.26.3.189-202.
Cite this paper
Irmak, N., Şiar, Z. & Keskin, R. (2019). On the sum of three arbitrary Fibonacci and Lucas numbers. Notes on Number Theory and Discrete Mathematics, 25(4), 96-101, DOI: 10.7546/nntdm.2019.25.4.96-101.


