Applications of Mollie Horadam’s generalized integers to Fermatian and Fibonacci numbers

A. G. Shannon
Notes on Number Theory and Discrete Mathematics
Print ISSN 1310–5132, Online ISSN 2367–8275
Volume 25, 2019, Number 2, Pages 113-126
DOI: 10.7546/nntdm.2019.25.2.113-126
Full paper (PDF, 153 Kb)

Details

Authors and affiliations

A. G. Shannon
Fellow, Warrane College, The University of New South Wales
Kensington NSW 2033, Australia

Abstract

This paper extends some of the arithmetic functions which Mollie Horadam developed for sequences of generalized integers and apply them to some particular integer sequences, particularly the Fibonacci and Fermatian numbers.

Keywords

• Fermatian numbers
• Lucas numbers
• q-Bernoulli numbers
• Divisibility sequences
• Ramanujan’s sum
• Möbius function
• Totient functions
• Co-prime
• Fibonacci numbers

2010 Mathematics Subject Classification

• 11B75
• 11Z05
• 11B65

References

1. Alter, R. (1973). Can φ(n) Properly Divide n – 1? American Mathematical Monthly. 80, 192–193.
2. Carlitz, L. (1948). q-Bernoulli Numbers and Polynomials. Duke Mathematical Journal. 15, 987–1000.
3. Carlitz, L. (1960). Some Arithmetic Sums connected with the Greatest Integer Function. Mathematica Scandinavica. 8, 59–64.
4. Carlitz, L., L. Moser. (1966). On Some Special Factorizations of 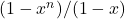(1–xn)/(1–x). Canadian Mathematical Bulletin. 9, 421–426.
5. Cohen, G. L. (1975). Selberg Formulae for Gaussian Integers. Acta Arithmetica. 16, 385–400.
6. Daykin, D. E. (1963). Linear sequences over a finite field. American Mathematical
   Monthly. 70, 637–642.
7. Hardy, G.H., E.M. Wright. (1965). An Introduction to the Theory of Numbers. 4th edition. Oxford: Clarendon Press.
8. Horadam, A.F., & Shannon, A.G. (1976). Ward’s Staudt–Clausen problem. Mathematica Scandinavica. 29 (4), 239–250.
9. Horadam, E. M. (1961). Arithmetical functions of generalized primes. American
   Mathematical Monthly. 68, 626–629.
10. Horadam, E. M. (1962). Arithmetical functions associated with the unitary divisors of a generalized integer. American Mathematical Monthly. 69, 196–199.
11. Horadam, E. M. (1963). The Euler φ function for generalized integers. Proceedings of the American Mathematical Society. 14, 754–772.
12. Horadam, E. M. (1963). A calculus of convolutions for generalized integers. Indagationes Mathematicae. 25, 695–698.
13. Horadam, E. M. (1964). Ramanujan’s sum for generalized integers. Duke Mathematical Journal. 31, 697–702.
14. Horadam, E. M. (1966). Addendum to Ramanujan’s sum for generalized integers. Duke Mathematical Journal. 33, 705–707.
15. Horadam, E. M. (1966). Exponential functions for arithmetical semi-groups. Journal für die reine und angewandte Mathematik. 222, 14–19.
16. Horadam, E. M. (1971). An extension of Daykin’s generalized Möbius function to unitary divisors. Journal für die reine und angewandte Mathematik. 246, 117–125.
17. Lucas, E. (1878). Théorie des fonctions numériques simplement périodiques. American Journal of Mathematics. 1: 184–240; 289–321.
18. Shannon, A. G. (2004). Some Properties of Fermatian Numbers. Notes on Number Theory and Discrete Mathematics, 10 (2), 25–33.
19. Shannon, A. G., &  Leyendekkers, J. V. (2014). Some Properties Associated with a Prime Characteristic Function. Proceedings of the Jangjeon Mathematical Society. 17 (2), 293–297.
20. Sloane, N.J.A., & Ploufffe, S. (1995). The Encyclopedia of Integer Sequences. San Diego, CA: Academic Press; Available online: https://oise.org/.
21. Williams, H. C. (1972). On a Generalization of the Lucas Functions. Acta Arithmetica. 20, 33–51.
22. Wooldridge, Kent. (1979). Values taken many times by Euler’s phi-function. Proceedings of the American Mathematical Society. 76 (2), 229–234.